HOW NERVOUS STRUCTURES HAVE IDEAS¹ [93]

W.S. McCulloch and W.H. Pitts

Introduction

Ever since Galileo started modern physics in terms of physical particles whose doings are known to our minds only by appearance, our study of the world has been divided between the physical sciences, including chemistry and physiology, on the one hand, and the mental sciences, like logic and psychology, on the other. The rift widened with the years. To us who have to work with the disorders of the nervous system and the consequent disorders of mental processes, the rift in science is obviously not in the patient, but only in our ways of thinking about him. Yet we have been unable to bridge the rift. In vain, Hughlings Jackson delineated the lesions of the brain which prevented propositionalizing. Physics cannot describe a psychological process. And Sir Charles Sherrington, near the end of a life well spent in studying the physiology of the central nervous system, was forced to the conclusion that “in this world Mind goes more ghostly than a ghost.”

In the last few years the art of communication has given birth to a new science. It has formed its central concepts and extended its domain to include brains as part of the general field of Cybernetics. In its terms, Mind is the circuit action to be expected of a structure like the nervous system. This bridge is common ground for psychology and physiology. Its program is to understand all purposive behavior, all that is truly functional, in terms of inverse feedback, and all sensation, perception, and ideation in terms of signals in appropriate networks.

We are all thoroughly familiar with inverse feedback in the form of reflexes — like the knee-jerk where the stretch we impart to the muscle causes impulses to pass to the spinal cord whence they are reflected to the stretched muscle and cause it to shorten.

We are equally familiar with the signals, for these are the all-or-none nervous impulses which pass a smoke ring of current to the axonal endings of any excited neuron. We know that the appropriate physiological stimulus for a neuron is the convergence upon it of simultaneous impulses in axons that end there. We know that there is a delay of less than a thousandth of a second between the time exciting impulses arrive upon a neuron and the time its impulse starts. Recently, thanks to David Lloyd, we know that the excitation of a neuron can be prevented by an impulse in an inhibitory axonal termination reaching the neuron about half a thousandth of a second before the impulses that would otherwise excite it (Fig. 1). As yet we do not know enough of the detailed structure of these junctions to distinguish them under the microscope, but we do know much of the general scheme of the connections of the net, for this is the anatomy of the nervous system.

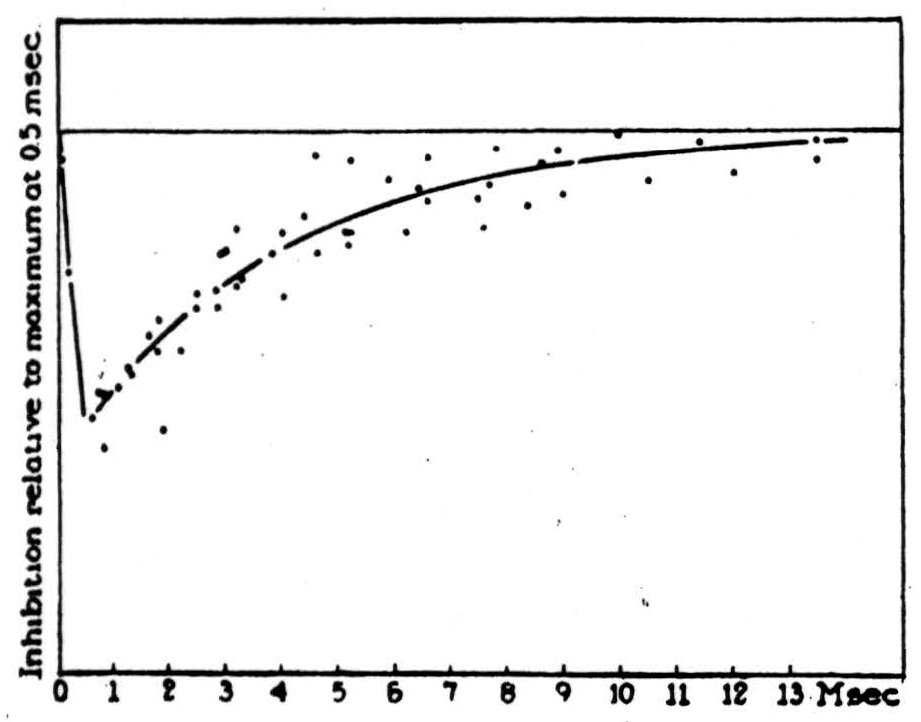
Figure 1.

Cybernetics is easy to understand if we get perfectly clear its central notion. All communication is achieved by signals. What is a signal? It can exist or not exist; and, if it exists, it is either true or else false. Physically, it exists as a thing which happens in space through time, and we can describe its physical properties. Thus we say of a given impulse that it occurred then in that neuron, and traveled from here to there at so many meters per second. That is half the story! It is the essence of a signal that it proposes something. A signal is true if what it says happened did happen, otherwise it is false — for example, paresthesia or hallucination. Thus a signal has the mental — the logical properties of a proposition. By definition, a signal is a proposition embodied in a physical process.

It is easy to think straight and speak clearly about anything if we have a clean, sharp language in which to describe it. This we can make by using for these embodied propositions the logic of molecular propositions and noting the time of each atomic proposition embodied in the impulse in each neuron. Since a nervous impulse happens only if that neuron was excited by impulses arriving upon it, what that impulse says is that the neuron was appropriately excited. A true nervous impulse implies that it was so excited.

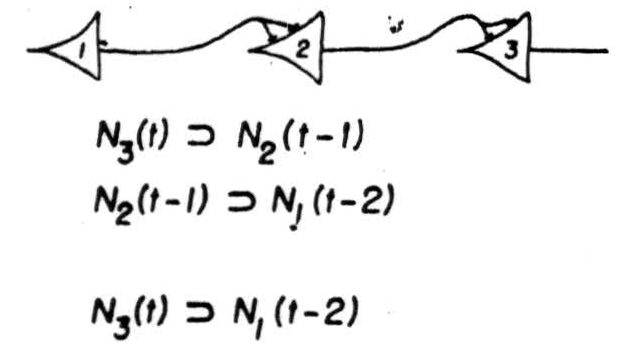
Figure 2.

Thus an impulse in Neuron 2, (Figure 2) Case 1, at the time 't’ implies the impulse in Neuron 1 at a time earlier by one synaptic delay. In one single case no information is lost; from the behavior of Neuron 2 everything about that case can be inferred. It is not so whenever Neuron 2 has more than one afferent, as in Figure 3, which enumerates the realizable cases for two afferents. We have exhausted all logical possibilities except for one; Neuron 2 cannot be made to fire when neither of its afferents does. In all of them there is a loss of information: positively, when, as in case 5 there are two possibilities, either of which may be responsible for its firing: negatively, in cases 2 and 3, where its failure to fire has no unique implication. Now loss of information always increases generality of some sort: in case 4, a signal on Neuron 2 implies only a general notion, where two instances are the firings of its two afferents, it is more particular. This calculus can be extended to more complicated nets. It permits us to construct a network between the receptors and a given neuron which makes the latter any desired logical function of the signals from the receptors at definite preceding times. There is an exception: we cannot make the output neuron fire when every receptor is silent; but a background of activity or a spontaneously active neuron removes this restriction. The output neuron embodies a general notion, the one whose instances are all complexions of signals from sense receptors sufficient to fire it. Its signal therefore implies that the world impingent on the sense organs was such as to produce these conditions.

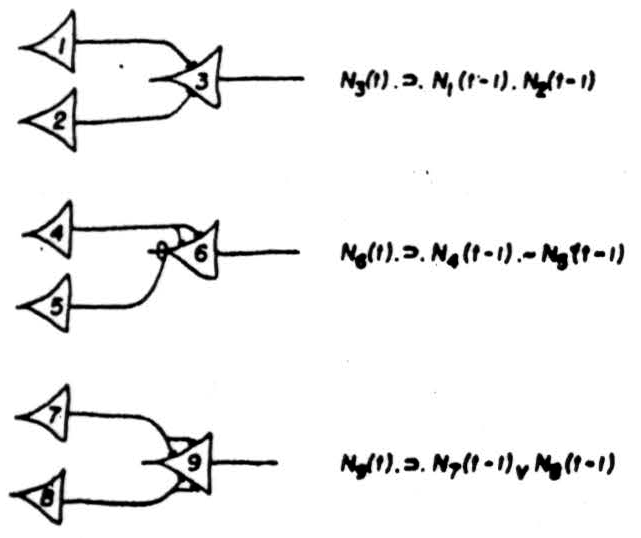
Figure 3.

Since nets can convert any figure given simultaneously in space into a figure in time, in a single place, and, conversely, any sequence in time into a figure in space, we cannot guess before hand what we should seek in the central nervous system when it is having a rudimentary idea. We must know the anatomy of the parts before we frame any hypothesis. But this we do know, that somewhere in that nervous system there must exist some invariant figure of activity every time that structure has that rudimentary idea. For example, there is no a priori reason to expect that each pitch picked up by the ear projects to a separate specific locus on Heschl's gyrus. Only the experiment discloses that to a given pitch corresponds an activity at a given place. Thus the place is the invariant of nervous activity which is having the idea - called “that pitch.” This invariant is a disjunct of the activity of the impulses from the two ears, for it comes to the same place no matter which ear picks up that pitch.

Figure 4.

Reverberation. (Figure 4). To get free of limitation of reference to one particular past time we seek an activity which as long (Figure 4). To get free of limitation of reference to one particular past time we seek an activity which as long as it lasts is the same no matter just when it was started. Such an activity is an active memory. Kubie and Ranson independently proposed that in the central nervous system there are closed paths. Around such a circuit, called a reverberating chain, activity patterned after some input may continue to circulate. At every round it has again the idea it received but once, hence it is invariant with respect to those times at one of which it was started. Lorente de Nó demonstrated the repetitive activity of the oculomotor mechanisms of the brain stem; and, for anatomical reasons, attributed it to reverberating chains. This activity is apparent in nystagmus. It may persist for minutes after the vestibular system has been excited by rotation. As long as it persists, that brain has the idea of that rotation, regardless of just when the rotation occurred. In short, it knows that there was some time at which there was rotation.

We have started from a rudimentary idea: “At the time 't’ the organism rotates,” and by applying what logicians call an existential operator to it; “At some time before Y the organism was rotated,” we have derived a general idea, “to have rotated.” This predictable is no longer an idea referable to only one event but a universal, for it is invariant for all events belonging to the group of past occasions. Given it at one time, the brain has made it for *all *times. Obviously any other form of memory, say one depending on structural change, will do the same for a brain, provided that the trace can be activated again; for the activity does not depend upon when the trace was made. To be a trace is to be a temporal invariant. But there is no a priori reason why a given memory must be preserved one way rather than another. Other considerations must guide our guesses and experiment decide between them. Yet of this much we can be sure that every kind of memory is a regenerative or positive feedback.

Inverse feedback. Inverse feedbacks, reflexes and similar circuits within the brain, also create invariants of nervous activity. Each reflexive circuit establishes some state of a system by returning the system toward that state whenever it is removed from it. Hence the state established by a reflex is the end in and of that operation. The retina adjusts slowly to total illumination so as to keep the excitation of its rods and cones more nearly constant. The pupillary reflex does the same thing more promptly. In the brain there are inverse feedbacks from the suppressor areas of the cortex via the nucleus caudatus and some lower structure to the thalamic nuclei, where they inhibit the relaying of impulses to the cortex. All three of these acting seriatim insure that the average total number of impulses reaching the visual cortex shall be invariant under changes of illumination from faint starlight to full sunlight. Thus a figure of activity in the visual cortex implies that there was some illumination at which the excitation of the retina was of that figure.

Superior Colliculus. There are inverse feedbacks which differ from reflexes because part of their path lies outside the body. We call them appetitive because they seek something beyond us. In engineering parlance they are said to be inverse feedbacks over their targets. One of the best known of these turns the eyes to look at anything which comes into the visual field. Cajal showed that its afferents arise in the eye, bypass the geniculate, and enter the superior colliculus by fibers turning up toward its surface. Julia Apter, while throwing a tiny spot of light into the retina and recording from the superior colliculus, mapped how the visual field projected upon it. Seeing it in transverse section, we find that the output of the superior colliculus, swooping laterally and to the rear from its lower layers, plays, partly crossed and partly uncrossed, upon the oculomotor system.

In Figure 5 we have superimposed two of Apter's schemes, one showing how the visual field maps on the colliculus — the other the places toward which the eyes point when that point on the superior colliculus is strychninized and the visual field diffusely illuminated. You will notice that the eyes turn in a direction and by an amount which would exactly center a point of light which would project to the strychninized spot on the superior colliculus. In short, it follows from the known anatomy of these structures and from Apter's experiments that the superior colliculus computes by how much and in what direction the eyes

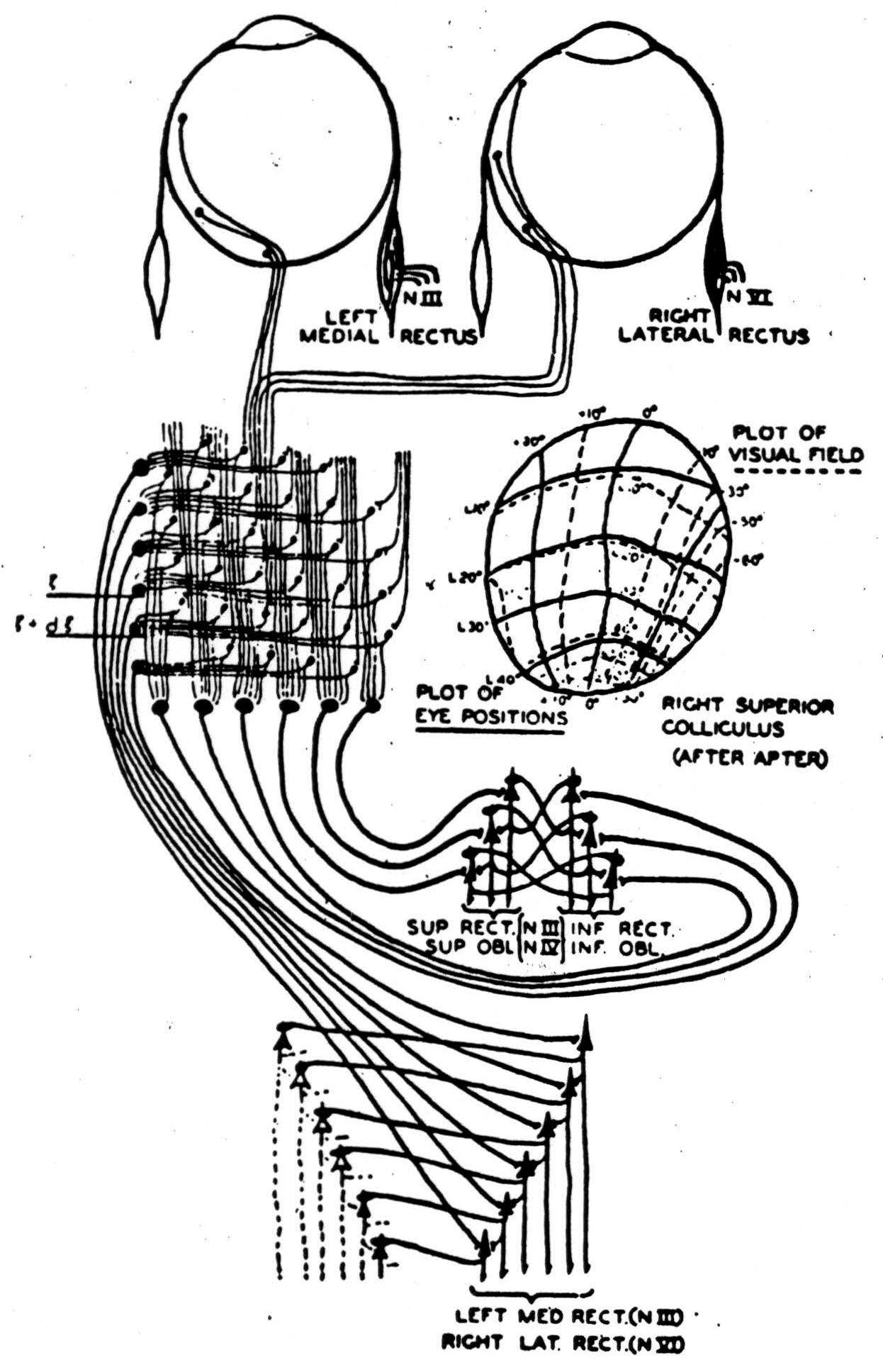
Figure 5.

must be turned to center a light appearing anywhere in the visual field; that it relays this information to the oculomotor nuclei, which then turn the eyes in the appropriate direction, so as to reduce the amount by which they must still be turned. When this amount becomes zero the eyes come to rest with this form centered. Patients with hemianopsia due to cortical lesions show this reflex when they are dark adapted and a light is placed in the blind field. I am told that if two lights are placed in the blind field the eyes turn to a position intermediate between them. If the cortex is intact they fix first on the one light, then the other.

Clearly, this circuit rids any visible thing of the gratuitous particularity of the position in which it first appeared. From all of its possible positions it has brought it to that predetermined one in which it is centered. This time our existential operator said: There was some visual location which was the location of that visible thing.

There is another way of having ideas. Consider the auditory cortex (Figure 6). It is a typical konio-cortex consisting of columns of more than a hundred small cells whose axons go straight down from the recipient layers to play upon the columns of cells below.

Figure 6.

To this cortex come auditory afferent fibers, so arranged that octaves span equal distances along Heschl's gyrus, with the low pitches coming to the outer and the high to its inner end; as these, the specific afferents, ascend through the recipient layers, they branch. Let us represent this schematically in Figure 7, where the incoming signals slant diagonally upward through the upper columns. Set the threshold of each neuron, a pulse to all cells of its layers and the incoming signal; and remember that the signal of that cell goes straight down. As we activate the layers serially the output will step off in the direction of the slant of the input - in this case to the right. Let us call this the axis of pitch - say, up the scale. Information slanting up parallel paths will be rigidly trans-

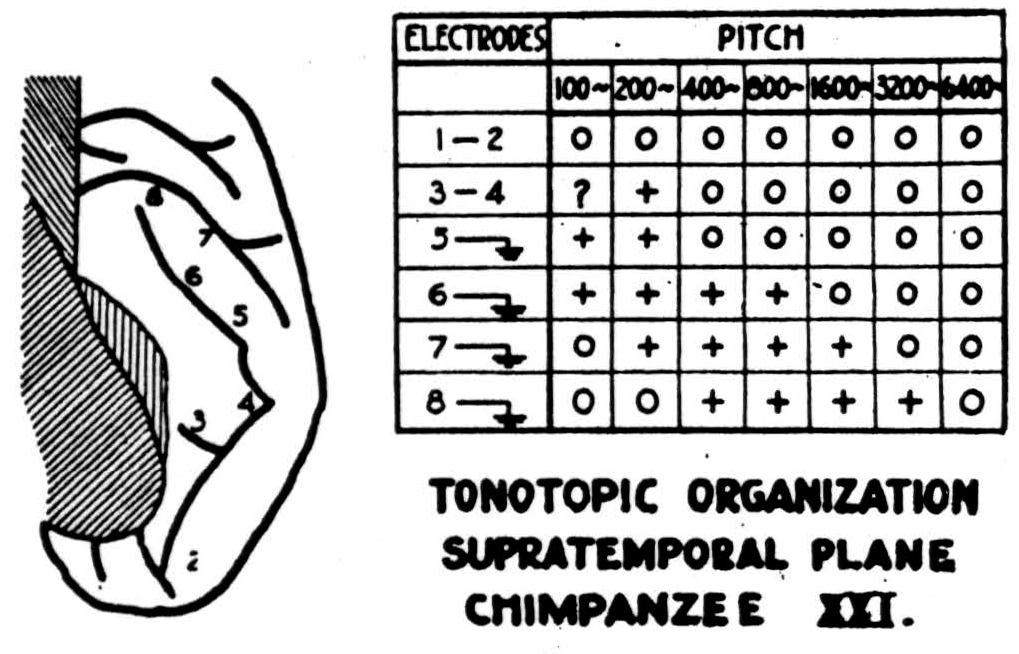
Figure 7.

lated in pitch with preservation of interval. Now, if we had an inverse feedback to stop this chord when it had moved up the scale to a preassigned position, we should have a mechanism very much like the reflex for centering forms to be seen. But there is nothing to suggest that we stop chords at one pitch. It seems far more likely that from a chord at one pitch we make the chord at all pitches, by exciting the recipient layers seriatim, and then add up the output in the lower layers. In a deeply narcotized monkey pitches project sharply to small separate loci. But in a chimpanzee so lightly narcotized as to have almost waking brain waves, although Heschl's gyrus is much longer, pitches produced surfacenegative waves that stretched the long way of the gyrus for about four octaves.

In primates the auditory cortex is a hard place to work, whereas the visual system (Figures 8 and 9) is easy and we know much more about it. Roughly speaking the fovea projects to the anterior margin of the area striata about half-way up its lateral margin, and it is the logarithm of the radial angle from the point of fixation that maps radially from this point on the cortex. Since the eyes turn to the center of the form to be seen, the visual cortex

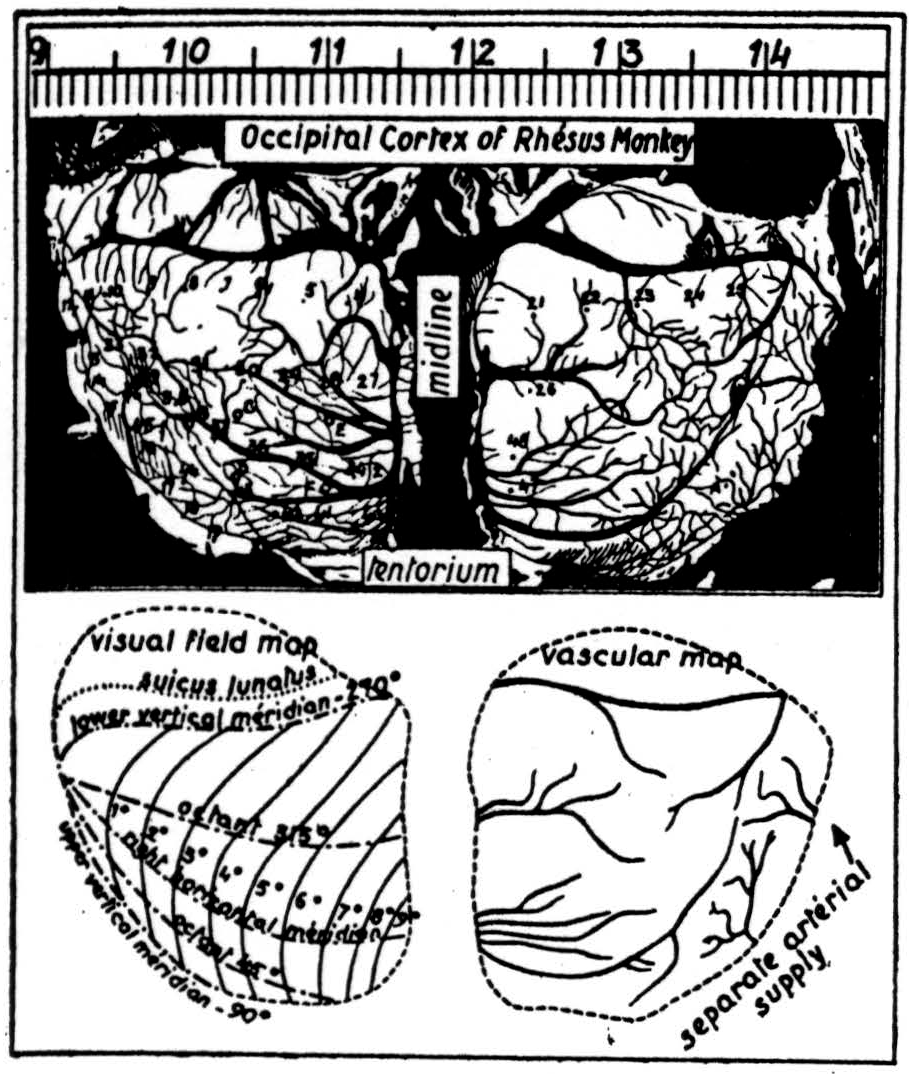
Figure 8.

does not need to do this; but it does have to see the same shape - regardless of size. Consequently, one would expect to find instead of the usual konio-cortex an enormous increase of sloping and horizontal branching of fibers from the geniculate. That is what makes area 17 the area striata. Again, we have among the branches layers of cells, becoming fewer as we ascend. The axons of these cells run chiefly upward to more superficial layers, which gather up the output to play on larger descending cells whose

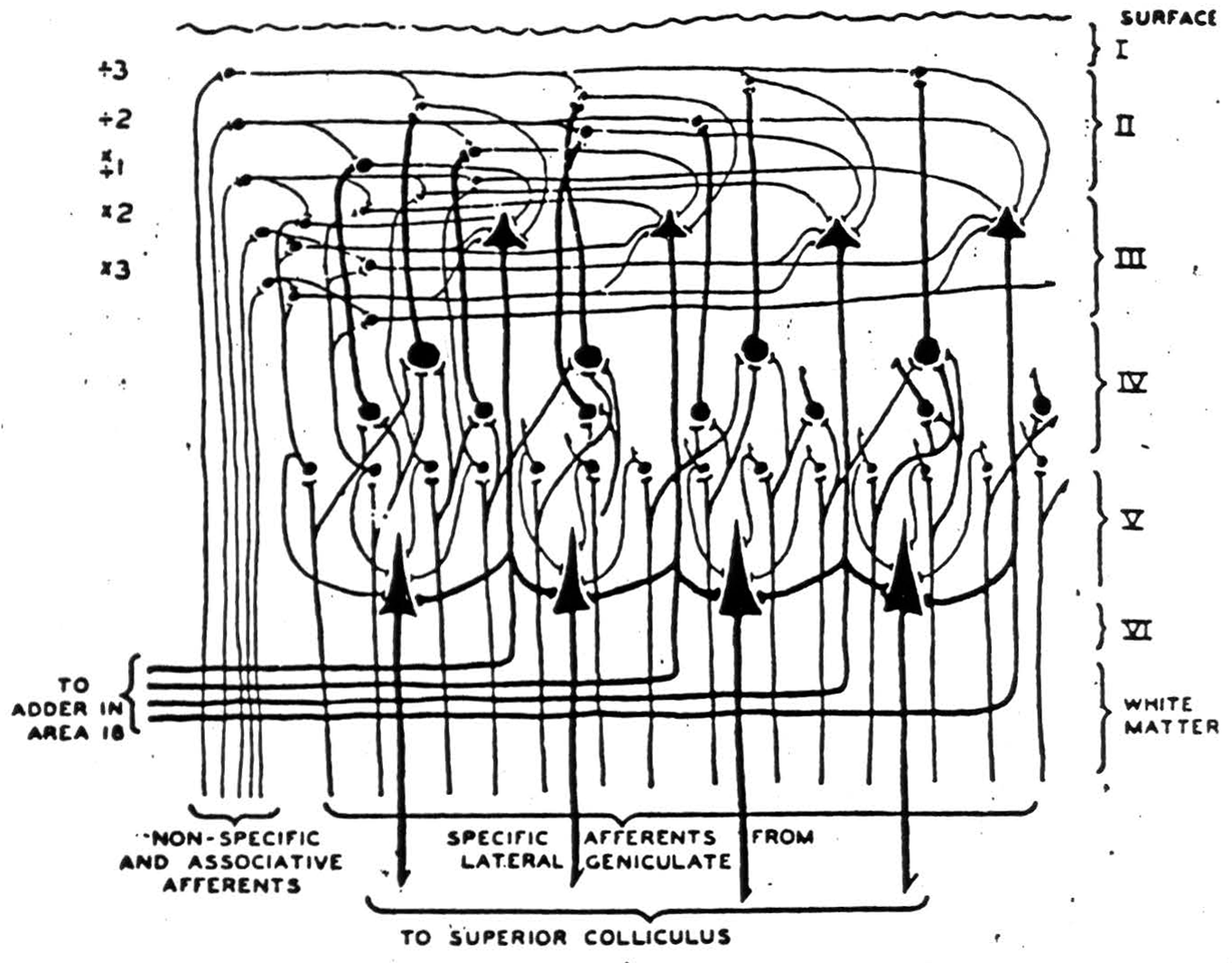
Figure 9.

axons leave this cortex. Now it is clear that if incoming signals follow axons branching toward and away from the representation of the fovea and layers are energized seriatim, then the output will move toward and away from the foveal representation while preserving its shape.

In short, if we were given a square of one size in the input we should have made squares of all sizes. It is established in the

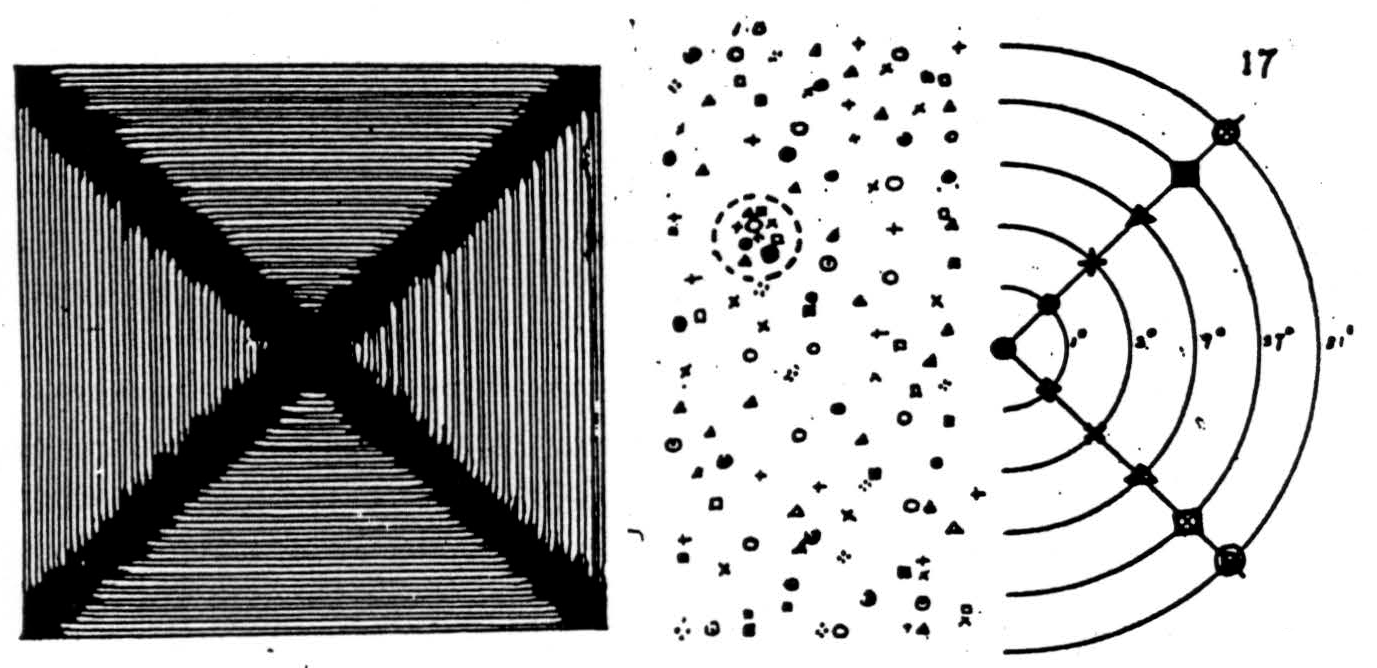
Figure 10.

psychology of perception, and supported by Talbot's analysis of the physiology of the jiggle of the eye in relation to on and off affects, that comers of squares produce far more cortical affect than do edges. Hence, the principal traces in the output of area 17 will be diagonal spokes produced by the expanding and contracting squares (Figure 10). Let us consider only these. When Dusser de Barenne and McCulloch placed strychnine anywhere in area 17, after it had time to soak down to the deeper layers and fire synchronously the cells there, they found typical spike potentials at scattered points in area 18 and even in area 19, indicating a random projection from any point in 17 to many points in 18 and 19. Now if a series of points is so strychninized, say along the spokes, it is clear that in areas 18 and 19 there will be some little region into which more will project than into any other. Consequently, an output from 17 along the spokes yields excitation maximum in this little region. Thus, from a square of one size in the input, the visual cortex makes squares of all sizes in the output, emphasizing the spokes and exciting this little region maximally. Consequently its activity defines a square regardless of size.

From this we can predict that faradic stimulation of a single focus in the striate cortex in a waking man will produce a visual hallucination, a blob of light somewhat like a sunset. It will be at a fixed point in the visual field and will move as he turns his eyes. That is what happens. Faradic stimulation of a point in area 18, and to a lesser extent in 19, should produce a report of a form seen, “a hand, a tree, a face.” It should neither be of a particular size nor of a particular position in the visual field. That is what happens. But there is a difference between a point clearly in 18 and one clearly in 19: in the latter case the form is seen as moving, in the former at rest, and at a position so well defined that the patient can turn his eyes to look at any part of it. We say “faradic stimulation,” for here, as elsewhere in the cortex, the parameters of stimulation — voltage, wave-form and number of pulses per second — probably play a significant role; and the old fashioned inductorium, with its fast, irregular waves, apparently produced much clearer hallucinations than our modem well-regulated devices.

You will notice that a circuit like this has several advantages, for the output is got by averaging the entire group of squares made from a single square, and the connections required for the circuit need not go to a particular cell but only to one in the right neighborhood. Even the threshold of the cells and the intensity of afferent bombardment may change much without appreciably affecting the outcome. Hence, except in the foveal representation, you may poke holes through the striata without changing the location of maximal excitation in 18. The holes might produce at least as big a scotoma as the optic stalk does at the retina, and the patient still see the form continuous through the blind spot. That is what happens. As a matter of fact, a patient with a homonymous hemianopsia, presented with but half a square in his seeing field, with top and bottom lines that extend to his blind field, ought to see a square. He does.

When we first came upon this hypothesis, we thought it would be very easy to check it histologically by the direction of branching of incoming fibers to area 17, for one would naturally expect they would have to run predominantly toward and away from the representation of the fovea. This is not so. Since it is not the radial angle but the logarithm of the radial angle that maps on the cortex, a circle on the surface of the cortex corresponds to an oval in the visual field with the little end of its long axis pointing at the point of fixation. Hence a very slight preponderance of radiality in the run of incoming fibers is all that one could hope to detect. For the rest, the visual cortex provides at least all of the connections proposed, as well as many others not necessary for this particular function.

One more point remains to be discussed. We have proposed that the layers of the cortex are alerted seriatim by what may be called a scanning sweep. For this argument it makes no difference whether that sweep has a rhythm intrinsic in the cortex, or is driven by the intralaminar and reticular nuclei of the thalamus, or is a reverberation between these two or between the cortex and its specific afferent nuclei, or is controlled by any combination of these. All that is necessary is to apply to the cells of the cortex something which alerts them, layer after layer, so that they then respond to the signals of their specific afferents. We should naturally expect whatever alerts the layers to appear as an electrical pulse in large electrodes on the cortex; and, because the axis of the cells of the cortex is predominantly vertical, we should expect it to lose its apparent rhythmicity the moment it goes to work; for the sweep of scansion would then disappear in the twinkle of the details comprised in the perception. This is exactly what happens to the alpha rhythm. Moreover, the 10 per second rhythmicity in the performance of the cortex has been known for many years. Probably Babinski, the physiologist, was the first who noted its importance in motor response to cortical stimulation, and found it could be accelerated to something like 13 per second and retarded to something like 7 per second before it broke to the half of double rhythm, he noted it as top frequency in the performance of music and the pronunciation of syllables. Ten per second is about the rate at which shapes can be seen regardless of size, and chords heard regardless of pitch. A man's alpha rhythm can be driven somewhat faster and slower by the specific afferents without disappearing entirely, but only when he does attend to the form or converge his eyes. Presumably, therefore, associational fibers help to alert the receptive areas — and that not diffusely, but in specifiable manners in the monkey and chimpanzee. On local strychninization the posterior portion of area 8 fires into area 18, and 18 fires into 17. Unilateral loss of area 8 causes pseudohomonymous hemianopsia.

Figure 11 is a record taken from Adrian's brain. He looked at the center of a flickering rectangle, and keeping his eyes focused there, attended to the margin, first on one size and then the other. As he did so, the alpha rhythm gave way to the winkle of details in the opposite hemisphere. Finally, to confirm the influence of the rhythmical activity of the brain upon its responses to incoming

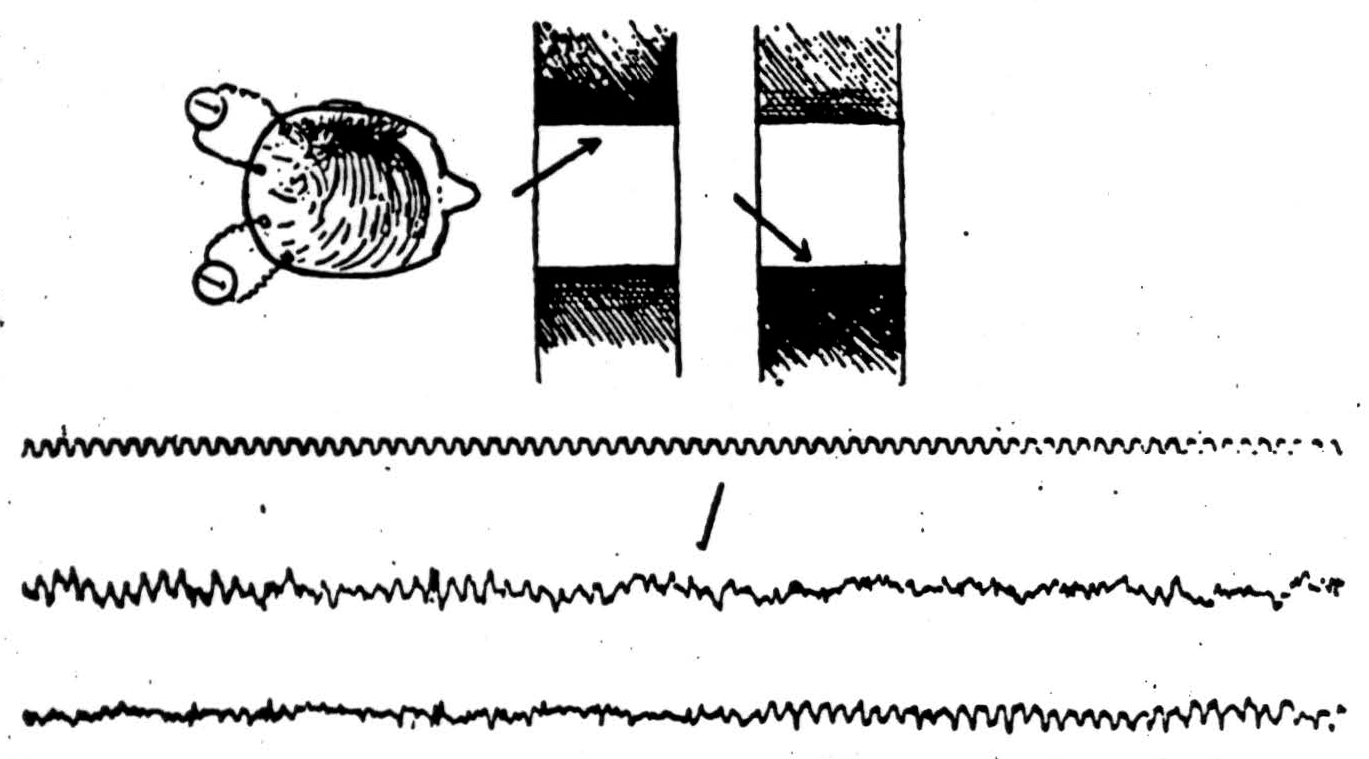
Figure 11.

signals, we may adduce Bishop's finding (Figure 12), that although the initial electrical signs of the incoming waves appear on the cortex, the cortex responds characteristically only during the subsequent phase of surface-positivity. The oscillations in excitability have a period of about a tenth of a second. We have seen the same thing in auditory cortex that he reports in the visual, presumably it may happen anywhere in the cortex. We await with interest the outcome of Teuber's experiments, combining the necessary psychological observations with electroencephalography the action of fever or thyroid or anything else that affects alpha frequency, to find out whether the relation is only coincidence of whether the alpha rhythm is itself the sweep of scansion.

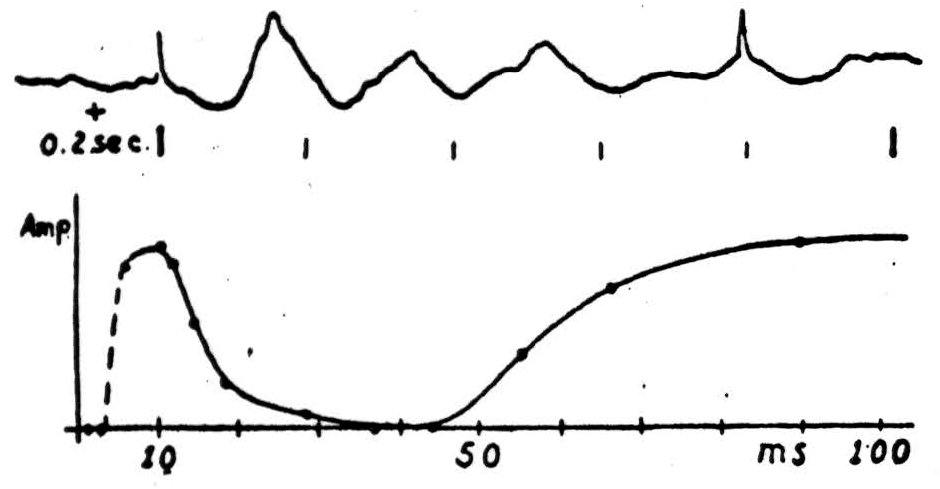
Figure 12.

Summary

Signals are embodied propositions implying their appropriate causes. Without memory or other circuits they can embody only rudimentary ideas. Reverberation and other forms of memory make ideas that are universal with respect to time. Reflexes, and similar feedbacks, by reducing some variable of the input to one among its many legitimate values give us universale with respect to the values of that variable; and nets that produce all the transforms belonging to some group, and then somehow average them, give us universale as invariants for that group of transformations -say shape regardless of size.

Footnote

For further research:

Wordcloud: Activity, Afferents, Area, Axons, Brain, Case, Cells, Center, Circuit, Colliculus, Cortex, End, Excited, Eyes, Feedback, Field, Figure, Fire, Form, General, Given, Happens, Idea, Impulse, Incoming, Invariant, Inverse, Layers, Light, Nervous, Neuron, Output, Particular, Per, Physical, Pitch, Point, Position, Produce, Project, Reflex, Rotation, Signal, Size, Square, Structure, Superior, System, Turn, Visual

Keywords: Structures, System, Particles, Physics, Sciences, Sherrington, Jackson, Cortex, Life

Google Books: http://asclinks.live/xb5x

Google Scholar: http://asclinks.live/mero

Jstor: http://asclinks.live/5hfz